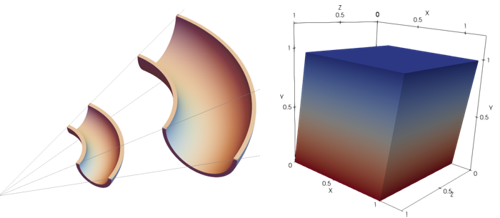
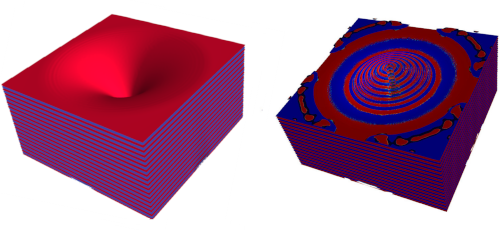
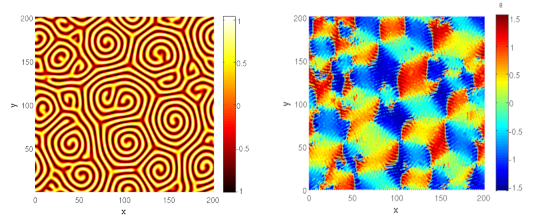
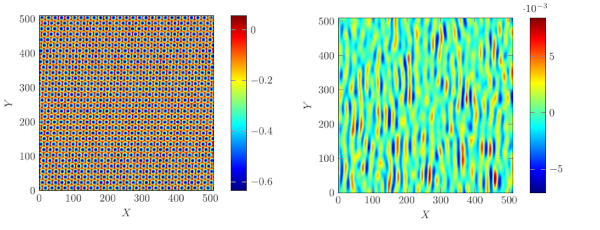
Quadratic-stretch elasticity, plate and shell energies
Crumpling on thin elastic sheets and inflatable surfaces are examples of systems where combined stretching and bending may appear. Commonly adopted two-dimensional energies present unfortunate mixing between these contents, such as the case of a plate whose midsurface stretches when a pure moment is applied. We formulate a quadratic-stretch elastic theory based on small Biot strains, from which plate and shell equations for arbitrary curvatures are derived. These present a separation of stretching and bending contents, for which a pure moment results in an isometry of the neutral surface. Our bending energies possess a number of desirable properties, including dilation invariance for a deformed plate. A quadratic-stretch energy also helps us understand issues with incomplete elastic energies such as the absence of Poynting effect for incompressible neo-Hookean materials under simple shear.